Nobody ever said that a card with an even number on one side would have a vowel on the other.

Photo Credit: WonderWhy/YouTube
I mean maybe you said that to yourself, but I never said that, and neither did Peter Wason.
Let’s go through the cards one at a time:
A: All of the most common answers, including the correct one, select this card. We know that if a card has a vowel on one side, then the other face must be an even number. In fact, that’s pretty much all we know.
K: There is no rule for cards with consonants that we know of. Most people seemed to get that concept and rightly ignored this card.
2: This is the dirty one that mixes most of us up. We don’t assume that K is matched with an odd number, but we do assume that even numbers have vowels, and that assumption is based in nothing. There is no rule about what’s on the other side of an even numbered card. So, there’s nothing to learn from it.
7: This might take a second to wrap your brain around, but the 7 is the ONLY other card besides the A that can help us either prove or disprove the rule. And either is fine. That’s another assumption we make: that the rule is true. We’re determining if it’s true or not. If the 7 flips over and it’s a vowel, that means that there’s a card with a vowel on one side that has an odd number on the other. That would disprove the rule.

Photo Credit: WonderWhy/YouTube
And the four cards were all chosen for a reason.
Each of them represent the four logical possibilities.
If we assume that this is an IF –> THEN statement, and IF=P and THEN=Q, this is what you end up with:

Photo Credit: WonderWhy/YouTube
So let’s get to the million-dollar question:
Why did most of us pick 2 instead of 7?
Remember that term from earlier: Confirmation Bias?
Confirmation bias is when we search out and prefer information that confirms what we already believe. Sometimes we ignore information that might disprove out preconceived notion, but often we will fail to even look for it.
Confirmation bias is essentially deductive reasoning. We start with an idea, come up with some way to prove that idea, and only look for evidence that confirms it.
Deductive reasoning is useful, but it also facilitates confirmation bias.
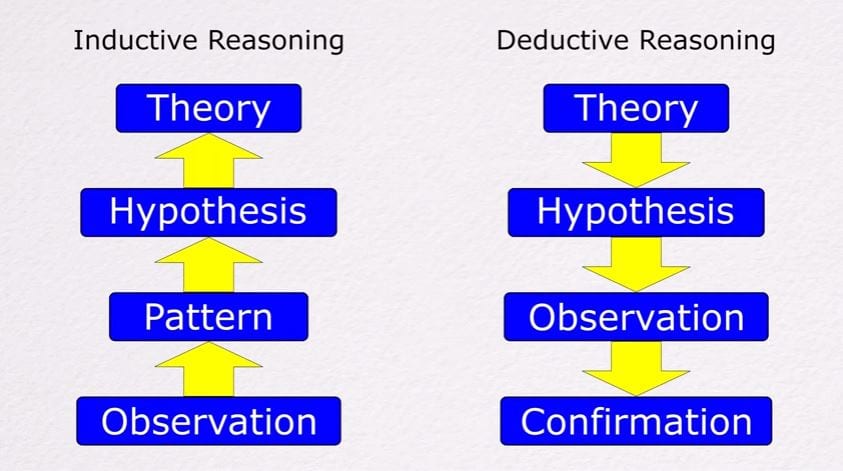
Photo Credit: WonderWhy/YouTube
Inductive reasoning is pretty much the opposite.
It starts with an observation, seeks out patterns that may exist, then forms a hypothesis to test, which becomes a theory.
You might remember those last two steps from a little thing called The Scientific Method.
Here’s the original video from WonderWhy:
If you want more, here’s another test, called the 2-4-6 task.
It was the first of Wason’s notable logic tasks, and it’s also a lot of logical fun:






